


|
Structural Engineering |

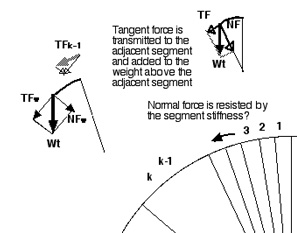 |
From right to left... Here each Wt is analyzed into its tangent force TF and its normal force NF.. The cumulation process is illustrated by analysing the k-1st segment´s tangent force TFk-1 which is then resolved into components that can be added to the Wt components of the kth segment, namely NFk and TFk... |
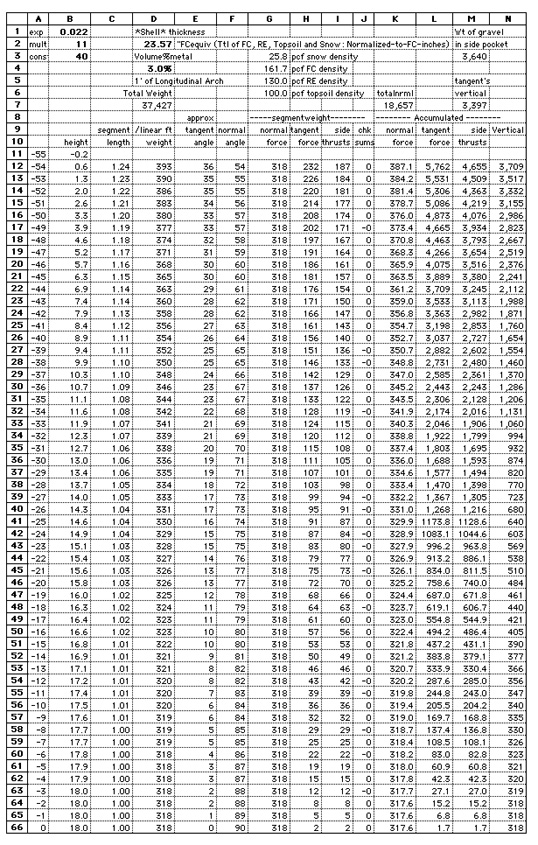
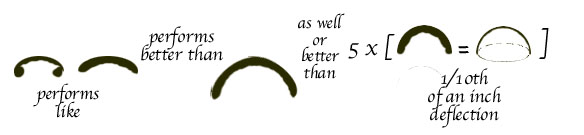
|
| Contact the
designer.
 A feature of
bergerac-tv.net
A feature of
bergerac-tv.net
|